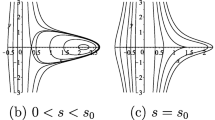
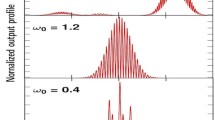
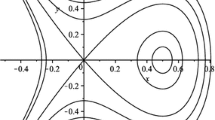
Abstract
By using biorthogonal decompositions, we show how uniformly propagating waves, togehter with their velocity, shape, and amplitude, can be extracted from a spatiotemporal signal consisting of the superposition of various traveling waves. The interaction between the different waves manifests itself in space-time resonances in case of a discrete biorthogonal spectrum and in resonant wavepackets in case of a continuous biorthogonal spectrum. Resonances appear as invariant subspaces under the biorthogonal operator, which leads to closed sets of algebraic equations. The analysis is then extended to superpositions of dispersive waves for which the (Fourier) dispersion relation is no longer linear. We then show how a space-time bifurcation, namely a qualitative change in the spatiotemporal nature of the solution, occurs when the biorthogonal operator is a nonholomorphic function of a parameter. This takes place when two eigenvalues are degenerate in the biorthogonal spectrum and when the spatial and temporal eigenvectors rotate within each eigenspace. Such a scenario applied to the superposition of traveling waves leads to the generation of additional waves propagating at new velocities, which can be computed from the spatial and temporal eigenmodes involved in the process (namely the shape of the propagating waves slightly before the bifurcation). An eigenvalue degeneracy, however, does not necessarily lead to a bifurcation, a situation we refer to as being self-avoiding. We illustrate our theoretical predictions by giving examples of bifurcating and self-avoiding events in propagating phenomena.
Similar content being viewed by others
References
V. I. Arnold,Geometrical Methods in the Theory of Ordinary Differential Equations, 2nd ed. (Springer-Verlag, Berlin, 1987).
N. Aubry, On the hidden beauty of the proper orthogonal decomposition,Theor. Comp. Fluid Dyn. 2:339–352 (1991).
N. Aubry, R. Guyonnet, and R. Lima, Spatio-temporal analysis of complex signals: Theory and Applications,J. Stat. Phys. 64:683–739 (1991).
N. Aubry, R. Guyonnet, and R. Lima, Spatio-temporal symmetries and bifurcations via biorthogonal decompositions,J. Nonlinear Sci. 2:183–215 (1992).
N. Aubry, R. Guyonnet, and R. Lima, Turbulence spectra,J. Stat. Phys. 67:183–215 (1992).
Aubry, P. Holmes, J. L. Lumley, and E. Stone, The dynamics of coherent structures in the wall region of a turbulent boundary layer,J. Fluid Mech. 192:115–173 (1988).
N. Aubry and W. Lian, Exploiting symmetries in applied and numerical analysis, inLectures in Applied Mathematics, E. Allgower, K. Georg, and R. Miranda, eds. (1993).
N. Aubry and W. Lian, Self-Similarity of in Transitional and Turbulent Compressible Flows, L. D. Kral and T. A. Zhang, eds., FED, Vol. 151, ASME 1993), pp. 53–60.
N. Aubry, W. Lian, and E. S. Titi, Preserving symmetries in the proper orthogonal decomposition,SIAM J. Stat. Sci. Comput. 14(2):483–505 (1993).
C. Baesens and R. S. Mackay, Uniformly travelling water waves from a dynamical systems viewpoint: Some insights into bifurcations from Stoke's family,J. Fluid Mech. 241:333–347 (1992).
G. Berkooz, P. Holmes, and J. L. Lumley, The proper orthogonal decomposition in the analysis of turbulent flows,Annu. Rev. Fluid Mech. 25:539–575 (1993).
G. Berkooz, P. Holmes, and J. L. Lumley, Coherent structures in random media and wavelets, in Proceedings of a NATO Advanced Research Workshop held in Estella, Spain, 8–14 September 1991, C. Pérez-Garcia, ed., “New Trends: Nonlinear Dynamics: Nonvariational Aspects,”Physica D 61:47–58 (1992).
F. Carbone, J. Liu, J. Gollub, N. Aubry, and R. Lima, Spatio-temporal analysis and bifurcations of wave patterns in flowing films, in preparation.
P. Coullet and J. Lega, Defect-mediated turbulence in wave patterns,Europhys. Lett. 7:511–516 (1988).
P. Coullet, S. Fauve, and E. Tirapegui, Large scale instability of nonlinear standing waves,J. Phys. Lett. (Paris)46:L787—L791 (1985).
R. Courant and D. Hilbert,Methoden der mathematischen Physik (Julius Springer, 1924).
A. Deane, G. E. Karniadakis, I. G. Kevrekidis, and S. A. Orszag, Low dimensional models for complex geometry flows: Application to grooved channels and circular cylinders,Phys. Fluids A 3:2337 (1991).
Y. Demay and G. Iooss, Calcul des solutions bifurquées pour le problème de Couette-Taylor avec les deux cylinders en rotation,J. Méc. Theor. Appl. (Spec. Iss.)1984:193–216 (1984).
M. N. Glauser, S. J. Leib, and W. K. George, inTurbulent Shear Flow 5 (Springer-Berlag, Berlin, 1987).
R. Grimshaw, Resonant wave interactions in a stratified shear flow,J. Fluid Mech. 190:3357–374 (1988).
J. Guckenheimer and P. Holmes,Nonlinear Oscillations, Dynamical Systems and Bifurcations of Vector Fields (Springer-Verlag, Berlin, 1986).
J. K. Hunter, Interacting weakly nonlinear hyperbolic and dispersive waves, inMicrolocal Analysis and Nonlinear Waves, M. Beals, R. B. Melrose, and J. Rauch, eds. (IMA Volumes in Mathematics and Its Applications, Vol. 30, 1991), pp. 83–111.
J. L. Joly and J. Rauch, Nonlinear resonance can create dense oscillations, inMicrolocal Analysis and Nonlinear Waves, M. Beals, R. B. Melrose, and J. Rauch, eds. (IMA Volumes in Mathematics and Its Applications, Vol. 30, 1991), pp. 113–123.
K. Karhunen, Zur spektral theorie stochatischer prozesse,Ann. Acad. Sci. Fenn. Ser. A 1 (1944).
T. Kato,Perturbation Theory for Linear Operators, 2nd ed. (Springer-Verlag, Berlin, 1976).
M. Kirby and D. Armbruster, Reconstructing phase space from PDE simulations,Z. Angew. Math. Phys. 43:999–1022 (1922).
P. Kolodner, C. M. Surko, and H. Williams, Dynamics of traveling waves near the onset of convection in binary fluid mixtures,Physica D 37:319–333 (1989).
J. Liu, J. D. Paul, E. Banilower, and J. P. Gollub, Film flow instabilities and spatiotemporal dynamics, inProceedings of the First Experimental Chaos Conference, S. Vohra, M. Spano, M. Schlesinger, L. Picora, and W. Ditto, eds. (World Scientific, River Edge, New Jersey, 1992).
J. Liu, J. D. Paul, and J. P. Gollub, Measurements of the primary instabilities of film flows,J. Fluid Mech. 250:69–101 (1993).
J. Liu and J. P. Gollub, Onset of spatially chaotic waves on flowing films,Phys. Rev. Lett. 70(15):2289–2292.
M. Loève,Probability Theory (Van Nostrand, New York, 1955).
J. L. Lumley, The structure of inhomogeneous turbulent flows, inAtmospheric Turbulence and Radio Propagation, A. M. Yaglom and V. I. Tatarski, eds. (Nauka, Moscow, 1967), pp. 166–178.
J. L. Lumley,Stochastic Tools in Turbulence (Academic Press, New York, 1970).
A. Majda and R. R. Rosales, Resonantly interacting hyperbolic waves, I: A single space variable,Stud. Appl. Math. 71:149–179 (1984).
W. I. Neuman, D. K. Campbell, and J. M. Hyman, Identifying coherent structures in nonlinear wave propagation,Chaos 1:77–94 (1991).
M. Reed and B. Simon,Methods of Modern Mathematical Physics I: Functional Analysis (Academic Press, 1980).
R. Z. Sagdeev, D. A. Usikov, and G. M. Zaslavsky,Nonlinear Physics from the Pendulum to Turbulence and Chaos (Harwood, 1988).
S. Sanghi and N. Aubry, Interaction mode models of near wall turbulence,J. Fluid Mech. 247:455–488 (1993).
L. Sirovich, Chaotic dynamics of coherent structures,Physica D 37:126–145 (1989).
L. Sirovich, M. Kirby, and M. Winter, An eigenfunction approach to large scale transitional structures in jet flow,Phys. Fluids A 2:127–136 (1990).
S. Slimani, N. Aubry, P. Kolodner, and R. Lima, Biorthogonal decomposition analysis of dispersive chaos in binary fluid convection, inBifurcation Phenomena and Chaos in Thermal Convection, H. H. Bau, L. Bertram, and S. A. Korpela, eds. (ASME, New York, 1992), pp. 39–46.
J. J. Stoker,Water Waves (Interscience, New York).
J. von Neuman, Charakterisiering des Spektrums eines Integraloperators,Actualités Sci. Ind. (Paris)229 (1935).
G. B. Whitham,Linear and Nonlinear Waves (Wiley-Interscience, New York, 1974).
P. K. Yeung, J. Brasseur, and Q. Wang, Dynamics of large-to-small scale couplings in forced turbulence: Physical and Fourier-space views,J. Fluid Mech., submitted (1993).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Aubry, N., Carbone, F., Lima, R. et al. Wave propagation phenomena from a spatiotemporal viewpoint: Resonances and bifurcations. J Stat Phys 76, 1005–1043 (1994). https://doi.org/10.1007/BF02188696
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/BF02188696